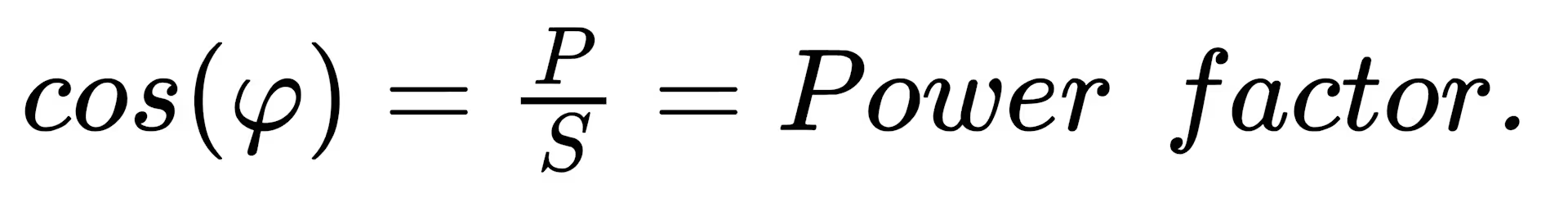Phase shift and reactive power
Reactive power is required to generate electromagnetic fields from machines such as three-phase motors, transformers, welding systems, etc. As these fields continuously build up and then decay, the reactive power oscillates between the generator and the consumer. In contrast to active power, reactive power cannot be used, i.e. converted into another form of energy, and places a load on the power supply grid and the generating systems (generators and transformers). Furthermore, all energy distribution systems must be designed larger to provide the reactive current.

It is therefore advisable to reduce the resulting inductive reactive power close to the consumer by a counteracting capacitive reactive power of the same magnitude as far as possible. This process is called compensation. During compensation, the proportion of inductive reactive power in the grid is reduced by the reactive power of the power capacitor or the compensation system (PFC). The generation systems and energy transmission equipment are thus relieved of reactive current. The phase shift between current and voltage is reduced or, ideally, completely eliminated with a power factor of 1.

The power factor is a parameter that can be influenced by network disturbances such as distortion or unbalance. It deteriorates with increasing phase shift between current and voltage and with increasing distortion of the current curve. It is defined as the quotient of the amount of active power and apparent power and is therefore a measure of the efficiency with which a load uses electrical energy. A higher power factor therefore signifies improved utilization of electrical energy and ultimately higher efficiency.

Power Factor (arithmetic)
- The power factor is unsigned

cos phi – Fundamental Power Factor
- Only the fundamental oscillation component is used to calculate the cos phi.
- Sign cos phi (φ):
- - = for delivery of active power
- + = for consumption of active power
Since no uniform phase shift angle can be specified for harmonic loads, the power factor λ and the frequently used effective factor cos(φ1) must not be equated. Based on the formula (Graphic 1)
where I1 = fundamental oscillation rms value of the current, I = total rms value of the current, g1 = fundamental oscillation content of the current and cos(φ1) = shifting factor, it can be seen that the power factor λ is only equal to the shifting factor cos(φ1) for sinusoidal voltage and current (g = 1). Thus, for sinusoidal currents and voltages only, the power factor λ is equal to the cosine of the phase shift angle φ and is defined as (Graphic 2)